33 Replies
@Gyro Gearloose
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.The answer
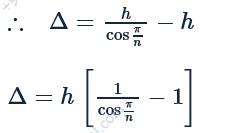
I'm having trouble visualizing this
The solution shows this diagram, I don't know what exactly's going on here
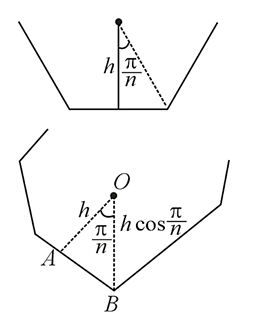
ye to olympiad ka hai yaar
lmao maybe
arey this is
properties of triangle
this makes sense
cuz look
Oh this looks fun
@Opt take over
mai bhi bhul gaya
:p
brother what i know how to take trig components
the diagram i dont understand
oh wait
i think i do?
hang on
Nimboi
Simple logic. The highest the COM is gonna get is when one of the vertices is on the ground.
So when the polygon has rotated by an angle of 2π/n
ah bingo
i got it
And like in the figure, half the angle rotated is gonna be π/n
Ok, now, mind if i give you a different question?
It was a fun one.
sure
Take a vertex of the rolling polygon, and consider its locus as the polygon rolls. What is the area under one loop(segment) of the locus?
This is a really fun result
huh
oke lemme try
Oh crap I forgot the proof myself
lmao
it seems to be tracing out an arc with the angle being the same as the angle of the polygon
(upon first rotation)
and on second rotation, it traces out the side of the polygon
my method doesnt seem very rigorous im just working w the example of a triangle and a square and seeing what results feel generalizable
Well, the topmost point is when the polygon stands on vertex. The height of the polygon-given h is the required height.
my method isnt exactly rigorous but is it
$\pi l^{2}\cdot\frac{\left(n-2\right)}{n} +$ the area of the shape itself
Nimboi [ping if answering]
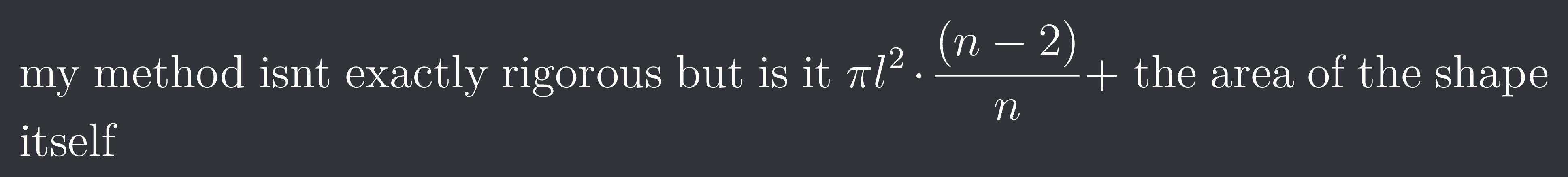
yuss i got that
opt proposed a new question
Uhhh I don't remember the formula, but i remember the result
Ah sorry.
It's (area of regular polygon)+2(area of its circumscribed circle)
2*area of the sector with radius
l (length of side) and angle of the shape + the area of the shape
area of its circumscribed circle as in?
are we saying the same thingOk it's
Area of regular polygon + $\frac{\pi l^{2}}{2}cosec^{2}\left(\frac{\pi}{n}\right)$
Opt

what 🧍
Just some basic trigo to figure out circumradius
Then 2πR²
so for a square it'd be
area + pi.l^2/4
i'm getting area + pi.l^2/2
hmpls ping me when u get a proper soln
