Electrostat
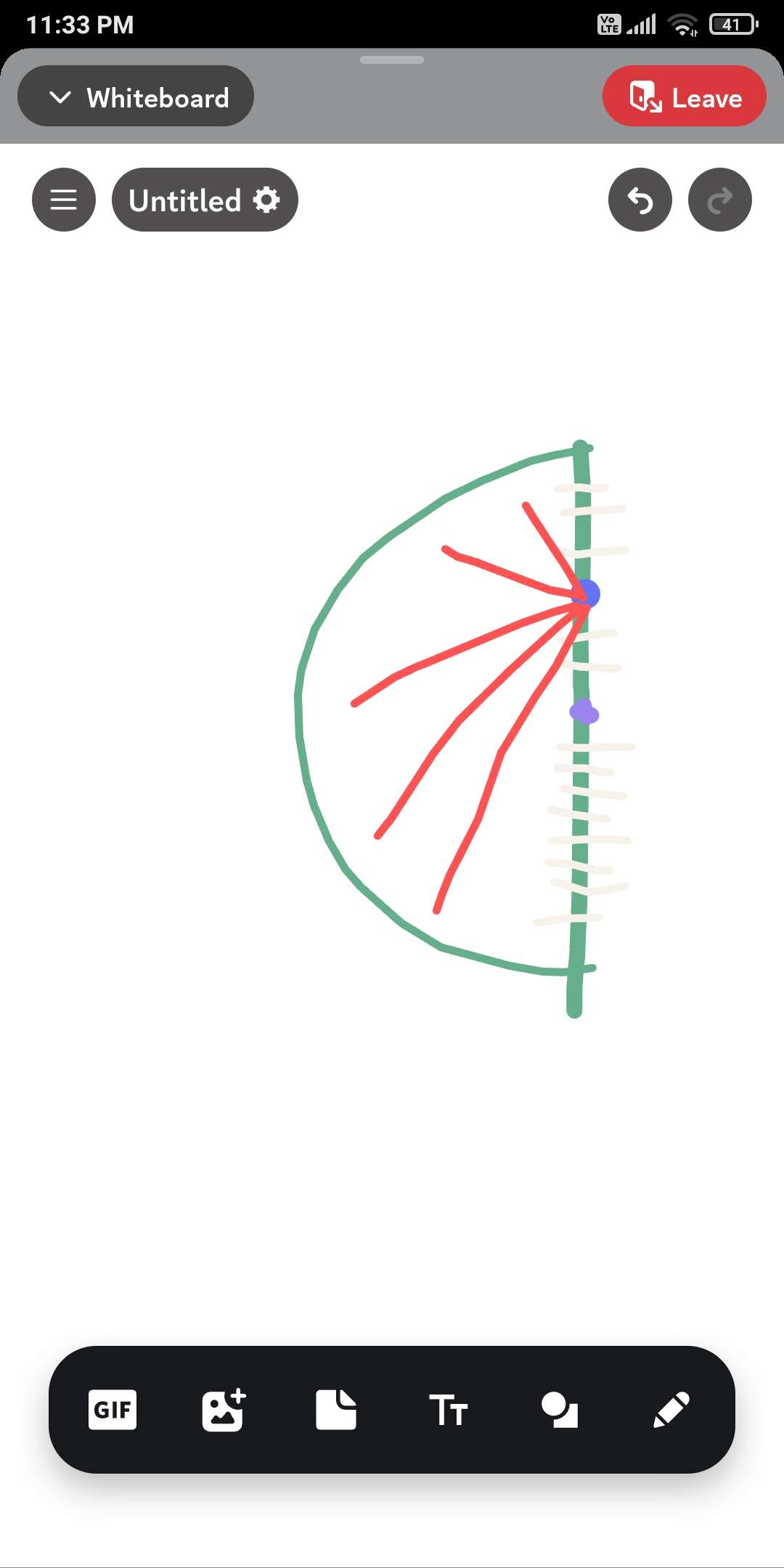
To ye semicircular ring h uniform charge density ki and mujhe jo blue pint h uske about nikalni h field. Lets say ki blue point ka altitude given
To fir iske upar field kese nikalenge. I thought of integrating the e vectors depicted in red but distance is also changing .
Could also look at it like a bended rod
12 Replies
@Gyro Gearloose
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.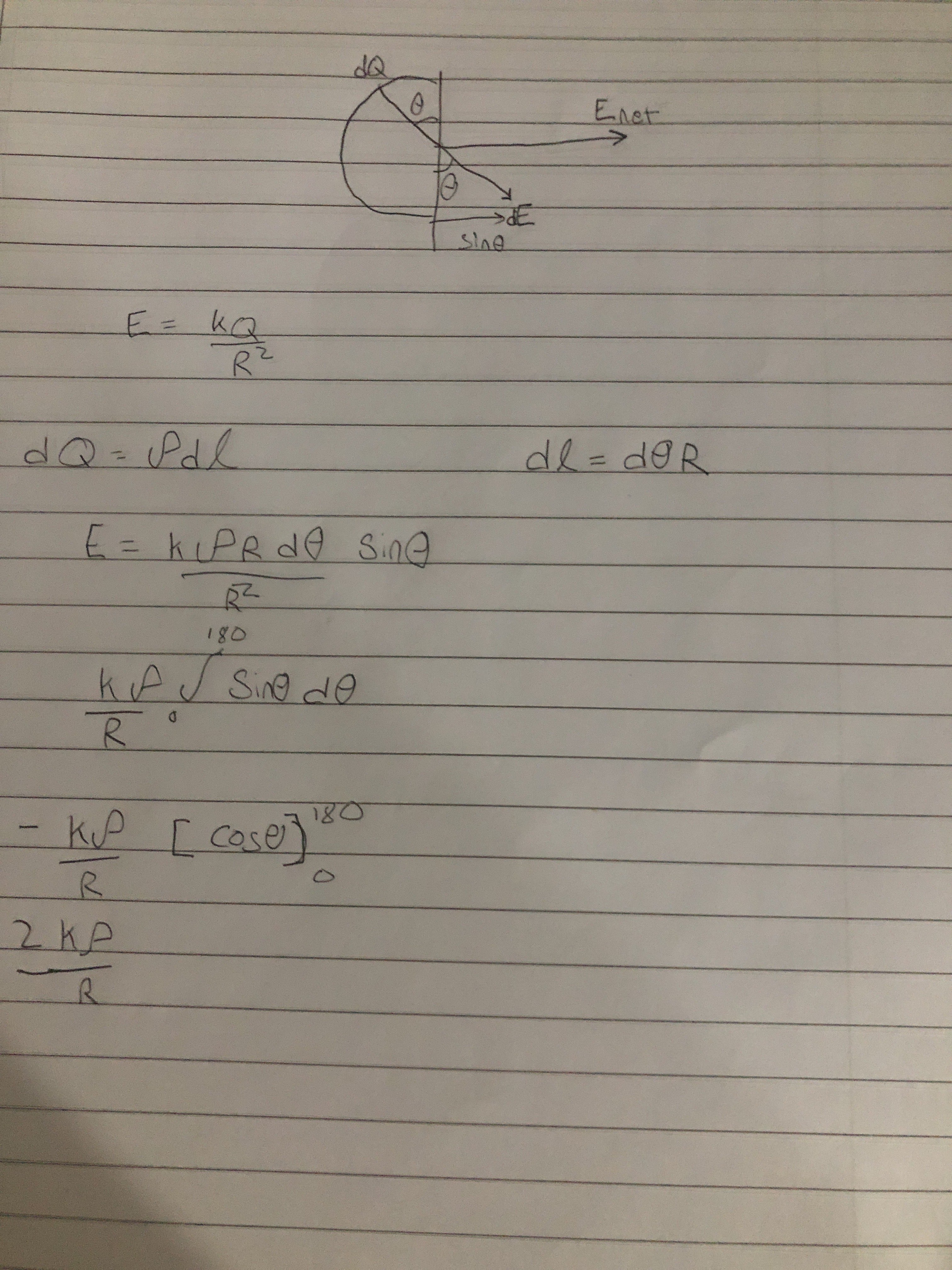
You can't integrate it like this, the distance between dq and particle is always changing
,rotate

The x component is turning out to be more troublesome
oh mb i thought you were talking about the center of ring
Yeah i tried that, its mind bending to say the least
I'm pretty sure it's going to be an elliptic integral with no elementary antiderivativr
okay so as u can see i found the distance x between point of observation and ring and found rest all using vectors and shit for electric field its js integration from 0 to pi of kdQ/x^2 and write dQ as lambda r * d(theta) (assuming like negligible thickness no surface area charge density and shit) and find out to components by doing sin(phi) for vertical (y component) and cos(phi) for horizontal ( x component) (i used some trig indentities to simplify phi) OH and also imp thing try putting in h=0 for a suprise
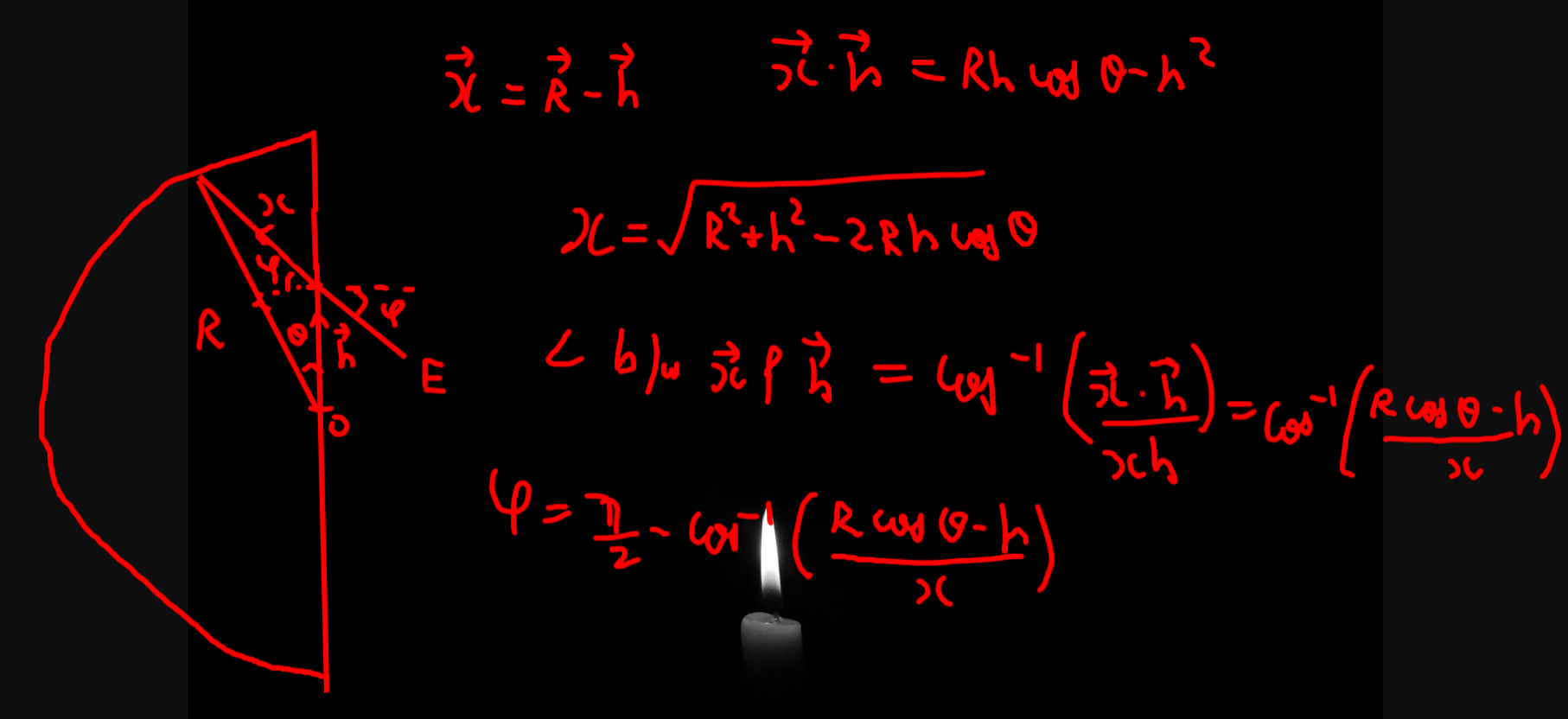
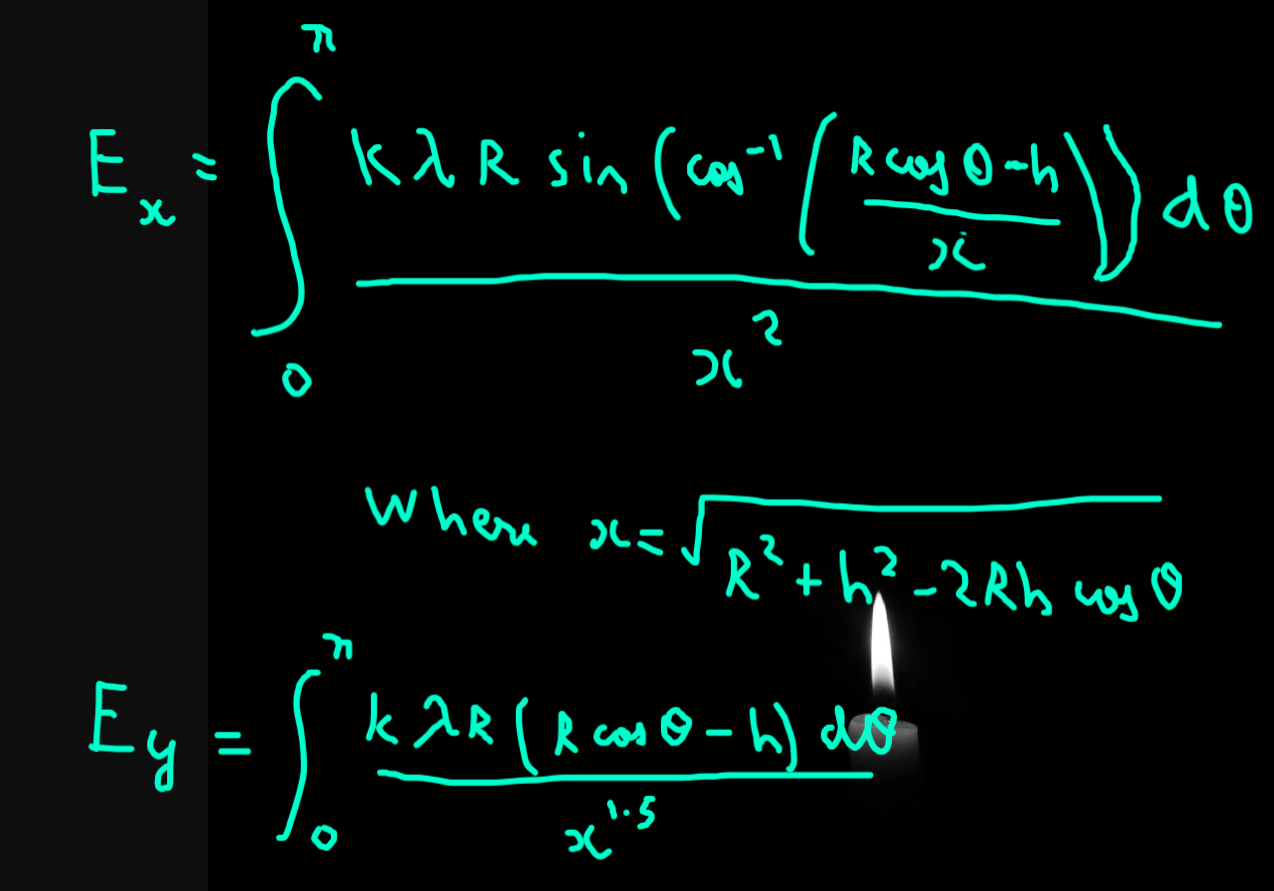
and those integrals are non solvable btw in this form only solvable for cases like h=0 or h>>R or h=R i think not sure u can check
btw this can be wrong too so ya