31 Replies
@Apu
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.ans is b ?
A,C according to key
for a concave upward curve to have 2 roots it should be decreasing first and then increasing so ththe derivative at two roots will be of opposite sign and let a b be roots of f(x) and then the you will get f'(a)g(a)f'(b)g(b)= some positive const since the llhs is a bi quadratic and since f'(a)and f'(b) have opposite signs g(a)g(b)<0 so it will have atleast one root
Um...should g(x) have any zeroes at all?
I honestly have no clue at all what the solution is meant to be. I wrote this paper just today.
I gotta write this out...

Okay nvm that's wrong.
Is there information given about g(x) being continous? Or can we prove the same here?
That is the entirety of the question. Nothing else.
I'm not sure about the first part, should g have exactly one zero? As for the c part, that is deducable. For the d part, consider some alpha<gamma<beta such that f'(gamma)=0. From the graph f(gamma)<0. Plug in the first equation to get g'(gamma)>0. Therefore at gamma+, both g and f are increasing, which is a contradiction to option (d).

Wait let me see if the solutions have been posted yet
*Cleare image
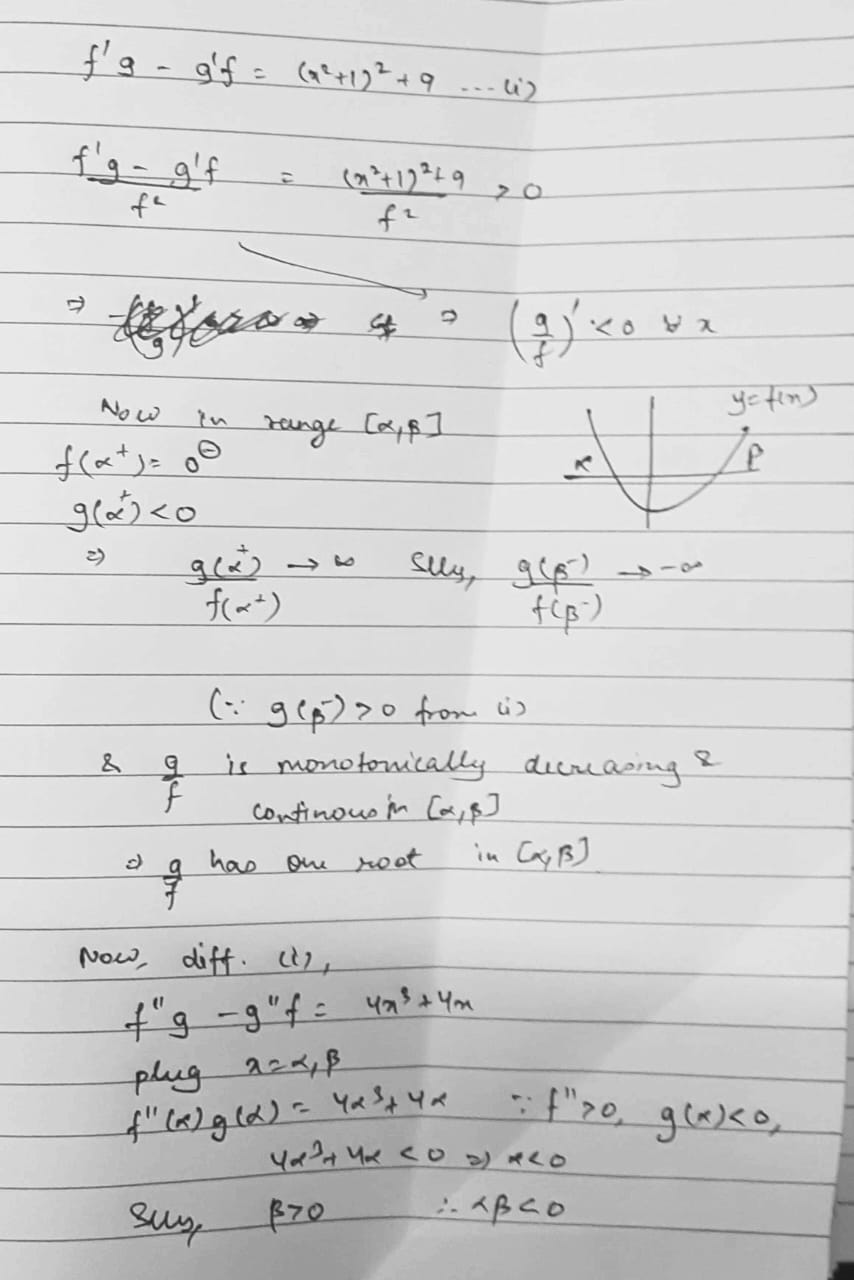
Ah. Do share if you find it.
I feel like I'm missing something here. How did they get that second derivative?

I'm confused
Wait solution says b, but the answer is given as a?
I think that's an error. They might have switched up the options while printing the question.
Looks like it's b,c
Ah.
It seems like they took it from a different spurce
*source
But from here, g/f is monotonically decreasing in alpha, beta, so shouldn't it have exactly one root?
I'll report an error if the window is still open. I don't think it is.
Also, mind if I send another doubt here?
Absolutely do.
The given answer is a,b,d but I got a,b,c,d by taking an example function (I chose a constant function of course)

Since the options are the most general cases, shouldn't it hold for any specific example?
Ig this is proving periodicity of function and stuff
I got that the period is 4, but it's annoying to use that logic
Yeah
So I took the straightforward approach of f(x) = 5/2 (from the given integral value)
Ah that works. (b) works because of the period being 4; there is symmetry about x=4n+2, so shifting the graph by even units doesn't change the area hence (d) works
(c) works only if there was some symmetry about 1 (which occurs when taking a constant function) and hence gives the incorrect result
Ig a counter example is abs(sin(pi*x/4))
Ah i see.
Welp, I'm screwed
+solved @SirLancelotDuLac
Post locked and archived successfully!
Archived by
<@763645886500175892> (763645886500175892)
Time
<t:1745470195:R>
Solved by
<@1075951732460376214> (1075951732460376214)
