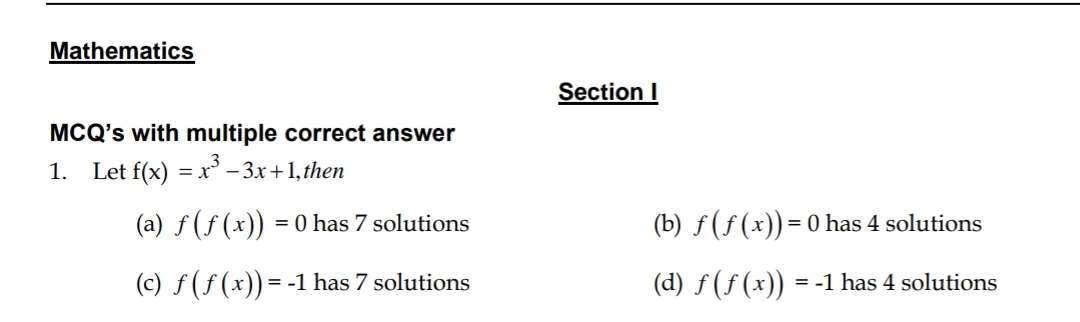
Differential calculus? Honestly, idk.
Q.1 and Q.15 had me stumped.
I forgot the method for depressed cubics so I couldn't even factorise the function in 1.
As for 15, I don't understand how we solve the DE with an inequality? If it was an equation, then characteristic quadratic gives us the solution, but with a ≥, I have no clue.
46 Replies
@Apu
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.Q.16 is also something I couldn't do, but that's a different topic, so, different thread?
For the 15th one, ig writing it as e^(-3x)(f''-3f')-e^(-3x)(2f'-6f)>=0 helps.
This would give e^(-3x)(f'-2f) is increasing. Since e^(-3x) is decreasing this means f'-2f must be increasing (monotonic).
1 seems okay enough?
graph banalo
or is it tough 😱
f(f(x)) ka graph?
yeah just calculate f(f(x)) then plot the graph
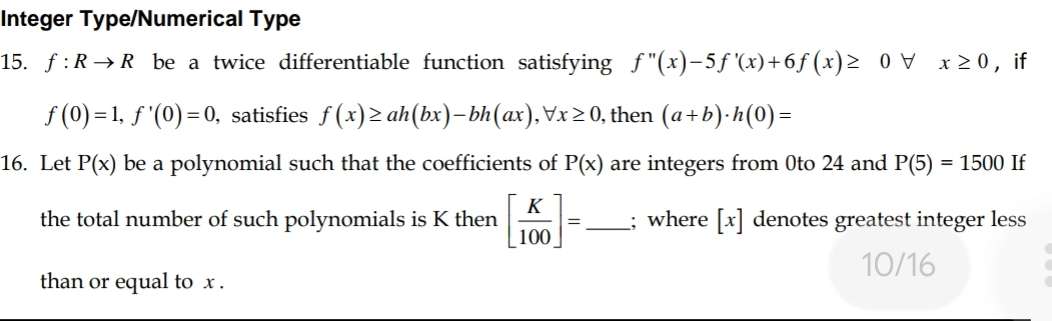
6 degree equation banegi
If roots are weird then idk what to do
f(f(x)) ke derivative ke roots nikalne honge
😿 tedious work
This is doable
Because f'(x) is factorable easily

yeah
actually
lemme tru
try
Oh ok
f'(f(x)) will change signs at -√3 0 √3 and 2
if f(fx) = 0 wala case lu to
yeah just calculate f(fx) then check consecutive points jahan dy/dx = 0 hai that f(fx1) f(fx2) is positive or negative
7 roots aajaenge
you have to check f'(x) also
there will be 2 more critial pts -1 and +1
then you will get 7 roots
question no 15 assume the function is Ae^2x+Be^3x (we take 2 and 3 because they are the roots of x^2-5x+6) and then satisfy f(0)=1 and f'(0)=0 conditions and then you will get f(x) to be 3e^2x-2e^3x so a =3 b =2 and h(0)=1
so ans is 5
I solved for f, but it's not equations, it's all inequalities. That's what confused me.
there is equality also right so it doesnt matter
16 one all i got is the degree should be 4 or lower i am not getting anywhere after this
Ok, that's just weird.
Yeah
It's going to be the no.of integral solutions of
625a+125b+25c+5d+e = 1500
Such that
0≤a,b,c,d,e≤24
In Q1, the minima of f(x) acquires a value of -1 rather than -3, so as for beta, there exists only one value of x such that f(x)=beta (Since beta<-1) [I made a slight error in the solution attached below.]
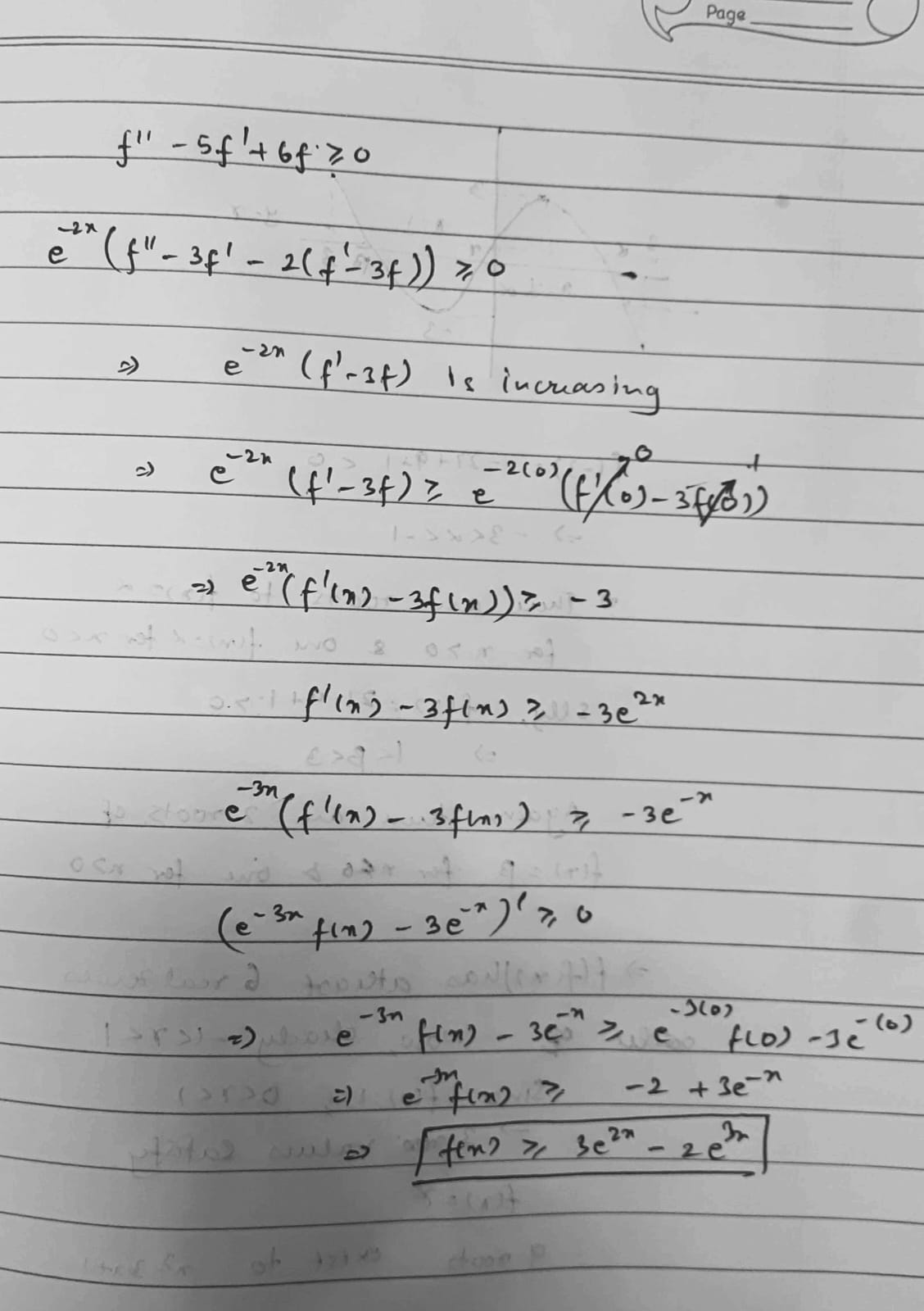

Ig (c) and (d) are by curve sketching of f(x)
Ah 16 would be a caseworking nightmare but here is something I thought of: Convert 1500 to base 25. Then 5a+b=(1500 in base 25) and we have info. about the number of digits in a and b. Does this logic work? :thinker:
I'm not sure if the above works, but just something I thought of.
Just a moment, why 5a+b? Wouldn't it be 25a+b?
Converting to base 25 covers positions of 1, 25 (the x^2 term), and so on but leaves out 5 (the x term), 125 and so on. Hence the multiplication by 5.
Nvm i understood
1500 = (2A0)_25
Where A denotes 10
2(625)+10(25)+0(1)
Ig we know that b must be divisible by 5, so considering it 5k would allow us to use multinomial ig.
b would be a 3-digit number while a would be a 2-digit one.
Rather messy, mb

@SirLancelotDuLac
When using multinomial ig we would have to do intermediate calculations in base 25 right?
To be on the safer side? Although that turns pretty hectic.
Um..Is the answer 1 for 16th?
It's given 3
Ah then I messed up :/
Wait, I hope the solutions copy is out.
I just have the answer key
Ig there are exactly 301 such polynomials.
Does this work?

Unfortunately, I don't know how to do maths in base 25. Let me try and decode it.
Neither do I lmao. I just tried to deconstruct and reconstruct the numbers from base 25 to 10 in my head.
Ig we dont need base 25 in the last 3 lines
Ok, where did you get the choice expression? (a+k)C1 ?
Beggar's method is not dependant on base (due to its derivation) so the expression remains same.
Ab ye beggar's method kya hota hai? :sweaty:
As for the value of the choice expression, that would also remain same due to its independancy from base.
The (n+r-1)C(r-1) thing. I heard someone call it beggar's method so I used it.
oh wait,
(n+r-1)C(r-1)?
I see.
Idk what anything's called honestly.
Oh, so we reduced it to no.of integral sols of a + k = 300
Yeah.
Well, if you have the solutions, do check if the value of k comes out to be 301.
Sorry, I'm still a bit confused. We got this expression, but how did we figure out the set of allowed values for a,k?
I think it'll be out around 7:30-8pm
The coeffecients of 1,x^2,x^4 are the digits of a. Since these can acquire values from 0 to 24, all numbers of upto 3 digits are valid.
Similar logic for b.
@SirLancelotDuLac

Yoo the method works 🙌
I mean, this one is more intuitive, but the base changing thing really shortens stuff out.
I still didnt understand the base change stuff 🤦♂️
Gimme a bit.
Yeah, I didn't explain it nicely. This is not a universal method, just seemed to work here. We consider a number of base 25 because the digits will give us the coefficient of the polynomial.
We have 625a+125b+25c+5d+e=1500
which can be written as (625a+25b+e)+5(25b+d)=1500
Let m be a base 25 number of the first bracket, and n be that of the second bracket.
Then we have m+5n=(1500 in base 25), since a,b,c,d and e can acquire values from 0 to 24, m and n can acquire values of upto 3 digit and 2 digit numbers.
Then let m=5k and apply beggar's method.
Again, not a general method 'cuz coeffecients might not always vary from 0 to 24 or like-wise, but still in this particular case shortens stuff out.
I see.
+solved @SirLancelotDuLac @hithav @Dexter
Post locked and archived successfully!
Archived by
<@763645886500175892> (763645886500175892)
Time
<t:1745592857:R>
Solved by
<@1075951732460376214> (1075951732460376214), <@726641475080683522> (726641475080683522), <@1095655769376034818> (1095655769376034818)